
Sunday, November 29, 2009
Tuesday, November 24, 2009
Wednesday, November 11, 2009
Tuesday, November 10, 2009
Enlarged Quadrilateral
Sunday, November 8, 2009
Enlargement 2

When you divide the area of the image by the area of the original shape the outcome is the enlargement factor multiplied by itself. This is proven the when the enlargement factor was 3, the answer was 9. With this information we can then predict that if the enlargement factor was 4 the area of the image divided by the area of the original shape would be 16, and if the enlargement factor was 5, the outcome would be 25.
Enlargement
Properties of Translation
Wednesday, November 4, 2009
Transformations
Tuesday, November 3, 2009
Triangle and Rotation Answers

From the triangle beside, we can see that both the original triangle and the rotated share that point which it was rotated on.
The actual shape remains the same (invariant) which means that the shape has been unaltered, merely rotated.
All the angles in comparison between the original triangle and rotated triangle were the same, there was no change in the angles post rotation. This can be proven by moving around the points of the original triangle and it's image will follow.
The lengths, like the angles, did not change upon rotation, they were also the same in comparison between the original and rotated triangles.
Angles are invariant

 under a rotation.
under a rotation.The lengths of the sides of an object are

 invariant
invariant 
 under a rotation.
under a rotation.The centre of rotation is the only
 invariant point.
invariant point.
Sunday, November 1, 2009
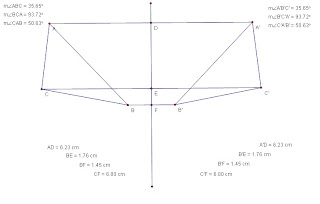
The distances of the reflected shape and the original shape remain the same from point to point.
No matter where are vertex is, the angles of both shapes are still equal.
 When animated, the distances between AD and A'D remain the same.
When animated, the distances between AD and A'D remain the same.All the distances in pairs (eg AD and A'D) are all the same.
 Angles are invariant
Angles are invariant  under a reflection.
under a reflection.The lengths of the sides of an object are invariant under a reflection.
Points on the mirror line are invariant under a reflection.
I have found that all angles and all distances and measurements were invariant between original shapes and reflected shapes everything was the same except that the shape was reversed.
Reflection: Triangle

The labeling system shows that when you mirror the object the labeled points are reversed.
When the mirror line passes through the base of the original triangle, the mirrored triangle is partially inside the original one
When a point of the original object is on the mirror line, it is called an invariant point.
Subscribe to:
Comments (Atom)


















